| g e n u i n e i d e a s | ||||||
 |
 |
 |
 |
 |
 |
 |
| home | art and science |
writings | biography | food | inventions | search |
| fire away |
|
January 2012
On a gas grill or electric stove, there's little challenge adjusting the temperature up or down. Simply turn the knob. But, with an open fire like this coal grill to the right, even dampers are ineffective. Air floods in from all directions. Other than spritzing with a bit of water, your only option is to move the food from the fire by sliding the coals aside or raising the grill. Sadly, most of us probably didn't pay too much attention during science class, but may vaguely remember hearing something about the "inverse square law". Which describes how the force of gravity varies with distance. As well as determines how much light intensity remains after traveling across a room or a galaxy. Since the food is not in contact with the coals in an open fire, and wind is blowing much of the hot air away, you are cooking with hot light- infrared radiation- and the inverse square law should apply. Or does it? To find out, we could perform a few integrals and solve the appropriate energy balance equations. Or, in the spirit of kitchen science, discover the answer experimentally with nothing more than a TV set and an old camera light meter. The "inverse square law" acquired its name from the governing equation, which states the power density at any distance "x" from a star or a candle follows this function:
Note the "x" is "squared", and located in the denominator (e.g., the "inverse"). Strictly speaking, this equation only applies to a one dimensional point source of light, not a flat tray of coals. But how far off can it be? It turns out, by a lot. Imagine you move the grating from 1" above the coals to 4" above the coals. By the inverse square law, the power would drop by (1"/4")2, or 94%! Anyone who has cooked over a grill knows whether you place your hand one inch above the grill or six inches, its still hellishly hot. So what gives? It turns out a flat source, if you are near enough, behaves nothing like a point source. Now, a single coal is not too different than a point source. In the illustration below, the red hot coal is emitting light uniformly in all directions. The colored rainbow is meant to convey the idea that the light intensity drops uniformly from hot (yellow) near the coal, to cool (blue) at a distance. And, the arrows schematically suggest beams of infrared radiation are leaving the coal. These arrows help us track the intensity of light at varying distances from the coal. For example, note five of the black beams hit the meat when it is close, but only three of these same beams (in white) intersect the meat at twice the distancei. Mimicking how power drops with distance.
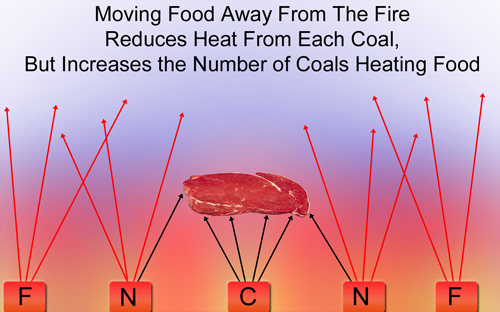
What happens when you replace a single coal with a tray of embers? Well, as you can see from the colors below, the formerly spherical light pattern begins to flatten out, as each coal fills in gaps in the radiation pattern. Also, like the single coal example above, as the meat moves farther away from the tray, fewer rays from the closest coal (C) hit the meat (dropping from 4 to 2), but now rays from nearby coals (N) take up the slack, and six rays still impinge on the steak. When the meat is removed further from the heat, N and C contribute fewer rays, but the far coals (F) now kick in:
Roll over the image to see how the number of heat rays impinging on the meat varies as it is moved away from the coals. Java must be enable for the rollover to function properly. Roughly speaking, when you pull the meat back by a distance "x" from the flames, the power of each coal drops by 1/x2. BUT, an increasing circle of coals (of about x2 in area) now brings their heat to the party. Almost exactly cancelling out the inverse square decline. In other words, when the meat is very near to a flat field of coals, its temperature does not depend on the distance from the fire! On the other hand, when the meat is much farther away from the coals than the tray of coals is wide, the thermal pattern looks more and more like a sphere, and it behaves like one large coal. Assuring a transition from no dependence on distance, back to the inverse square law.
If you are interested in the math, see the footnote (ii) below. The correct equation for the power density from the center of a flat, radiating plate is approximately:
(where in this case "x" is the ratio of the food's distance from the coals to the width of the coal tray). But, instead of math, we can discover this relationship experimentally, and in only five minutes. Rather than working with a hot tray of coals, any flat light radiating surface should do. Light is light. And most of us own a flat light emitter in the form an LCD televisioniii. I hooked our TV up to a laptop, and projected a square white image on the screen that was about 22" on a side. Then, I took an old camera light meter and a tape measure, and recorded the light levels at different distances from the screen. The experimental plot is shown in RED below: roll over image to compare the measured light intensity (red curve) to theory If you roll over the image, you can see how well this measurement agrees with the inverse squared law (green) and the Flat Plate Model (blue). The FPM is extraordinarily close to the measurements, so close the blue line is mostly hidden by the red experimental data. The inverse squared line only fits the data out beyond a distance of 1.5 times the coal tray width. For those of you still a bit flummoxed by all these equations and calculations, here is a real world example. Imagine you fired up a 24" diameter bed of coals (like many a grill). This curve illustrates how the heat flux varies with distance. Again, whether you are 1", 2", 3" or 4" above the coals, the heat flux (and thus the temperature) hardly varies. When you are 24" above the flames, the heat flux has dropped by around 60%. Real grills are pretty limited in the distance they can put between the meat and the fire. A 30" wide grill rarely offers a shelf 24" above the flames. Depending on how much air cooling the food receives away from the heat, the color of the grill (which affects how much light is absorbed), and so on, you have much less temperature control over an open fire than on a gas burner. So most cooks have learned to rake the fire away from one corner, creating a "safety zone" of low heat. Moving the food above the heat is much less effective at reducing temperatures than sliding it to the side into a region with no radiation at all. Just as this experiment demonstrates. A lot of trouble? Yes, but the flavor is worth the effort. |
|
--------------------------------------------------------------------------------------------------------
i Light ray tracing in a useful fiction, but glosses over two important physical phenomena. First, there are no actual individual beams emitted from coals, but a continuous flow of radiation that we divide into arbitrary bundles of solid angles and call them "rays". More importantly, rays from a distant light source impinging at a glancing angle to the food carries very little energy. Which is why we orient a solar panel perpendicular to the light, or face into the sun to warm ourselves on a cold winter day. This is why our drawings concentrated on those rays arriving closest to perpendicular on the food. Never the less, with a little practice, ray tracing offers realistic insights into the behavior of complex electromagnetic fields. ii A clear derivation of the radiation emitted from a flat circular plate can be found here, or in most first year physics texts. There is also a complex nomograph in Modernist Cuisine 2-16. iii In the early days of LCD televisions, the screen only emitted light in a narrow forward cone. Which meant if you sat to either side of an early LCD, the image was either dark or color-blurred. Fortunately, newer TVs offer nearly ideal, uniform flat plate lighting, and every seat in the room has a great view. |
 Contact Greg Blonder by email here - Modified Genuine Ideas, LLC. |