| g e n u i n e i d e a s | ||||||
 |
 |
 |
 |
 |
 |
 |
| home | art and science |
writings | biography | food | inventions | search |
| just a passing phase |
| Dec 2023 |
|
Walls are such a pain. Keeping people out. And in. Not too surprisingly, humanity has always wished for a power to walk through walls. From the bible, to ghosts making an eerie appearance late at night, to Superman1 and the Flash vibrating and "phasing" through concrete. How can we pass one solid body through another? Or at least a plane through a plane? For simplicity, let's combine these two cases into a single mechanical engineering challenge- passing a solid through a plane. Like a cube through a wire fence.
An unsuccessful phasing Solids, of course, feel solid but are nothing of the sort. Everything is made from atoms, and the constituents of an atom (electrons, protons and neutrons) are vastly smaller than the intervening space. In some minerals, like polymers and organic crystals, there are even pores between chains of atoms2.
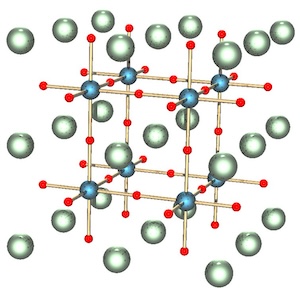
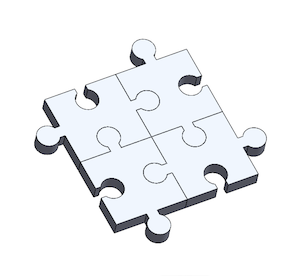
The perquisite crystal structure. The most abundant mineral in the Earth, bridgmanite, has this structure. Its chemical formula is (Mg,Fe)SiO3; the red spheres are oxygen, the blue spheres silicon and the green spheres magnesium or iron. So for a mechanical analog of phasing through a wall we will fabricate a wire mesh as the wall, and an array of "rods and atoms" as the solid. Then magically the solid's atoms will connect and disconnect as the cube encounters wires in the mesh. But is this possible? In fact, we are surrounded by structures which appear solid but are really a meshed-array of independent units ("tiles"). For example, a jigsaw puzzle looks like continuous mat, and if you are careful, a "fully interlocking puzzle" can be lifted into the air as one continuous sheet. Essentially, the puzzle is an array of independent tiles each captured by their neighbors via tabs called "interjambs" that snap into slots called "blanks". Topologically though, you could freely pass a thin wire from one edge of the puzzle to the other.
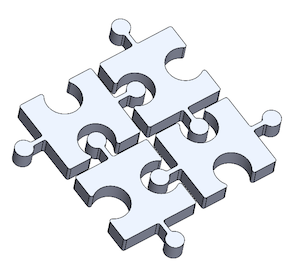
Tightly snapped jigsaw tiles. The same connection principle applies in 3D Now imagine we shrink the tiles, opening up an alleyway to ease a wire rod through the maze:
Gapped jigsaw. A wire mesh could easily slide down the alleyways from one edge to the other This jigsaw would fall apart, unless the gap is somehow filled with a material which maintains spacing, yet allows a wire to pass with little friction. One possibility is to fill the alley with opposing bristles. Bristles are stiff when compressed (so the alleyway doesn't collapse) but flexible in bending. Assuring the wire can move unimpeded. Like this brush grommet:
Still, friction may be an issue. Magnets! Why not insert repelling magnets to maintain the gap? Sadly, it turns out Earnshaw's Theorem proves any static array of floating magnets is always unstable. The narrow alley will slam tightly closed, blocking the wire. With enough force the wire might squeeze by3, but we can do better. One possibility is a zipper. Like the jigsaw, a zipper appears as if it connects one side of a raincoat to the other seamlessly, but in fact a thin wire could slide from top to bottom like floss between the teeth.
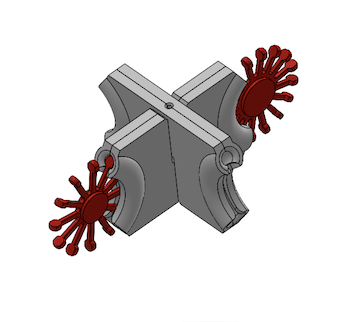
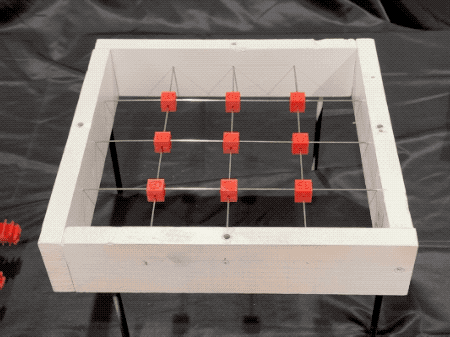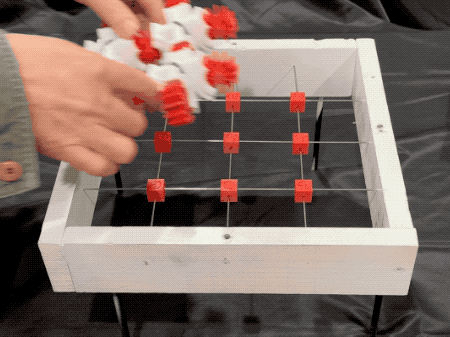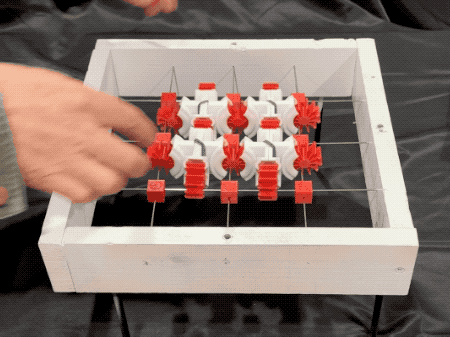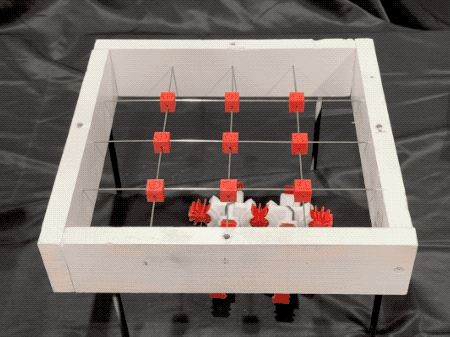
We can morph a zipper onto a disk, so the teeth connect and disconnect as the disk turns, yet the zipper mechanism prevents the disk's axles from changing their center-to-enter distance. Maintaining an open alleyway. Here is one such design: A few iterations of the zipper turnstile indicated friction is unacceptably high. What if we fill the alley with tiny turnstiles, to prevent collapse but rotate freely as the rod passes? That might work, providing we can the turnstile spacing remains fixed, without blocking the alley. So the gap is maintained. Thus we pivoted to this very simple alternative. A spoked turnstile with tabs on the end. These tabs are captured by a slotted channel, allowing rotation but no translation. Resulting in a simple tiled element, not too different than the jigsaw puzzle pieces above with two interjambs and two blanks.
And snapped into an array: The prototype was fabricated on an FDM 3D printer (which extrudes plastic in thin layers). Worrisomely, the layers acted like ratchet teeth, interfering with the sliding motion. But a little light sanding and adjusting the build-direction so the layers were oriented in the direction of motion avoided binding. Friction was acceptable. A quick perspective on the working mechanism: And a version with a wider turstile for stability:
Other approaches are possible, and likely superior. Genetic engineering and molecular assembly has advanced to the stage of prescriptive design. Nature may already rely on a zipper mechanism for phagocytosis, where a cell transports a large particle through the cell wall. And as the video mentions, DNA replication and translation, along with cell motility, are facilitated by marvelous DNA machinery which would put any modern factory to shame. |
|
1 Actually, this project was inspired from the world of math, and not the universe of superheroes. There is a wonderful short film animating the "eversion" of a sphere, and I wondered if a physical demonstration of eversion were possible. But this would require building a planar sheet that could phase through itself. In this case the rods would be elastic so the plane could bend and twist. 2 The ball-and-stick model of crystals is only meant as a notional guide to sold-state structure. In fact atoms are really extended quantum mechanical fields that suffuse "empty" space. Balls and sticks are a useful pedagogical tool, but overly simplified. Unlike our mechanical model, if you tried moving atoms through the empty space in a solid, their fields would interact and either block or distort motion. Even a pore-filled mineral like a zeolite might admit a few strays atoms into the pores, but not an entire solid. 3 Magnetic tiles can link together, producing a cubic lattice with snap-apart alleyways (magnets which touch or are held in place with a rigid framework do not violate Earnshaw's Theorem.) We experimented with cylindrical magnets forming a rotating seal, and turnstiles. The best design was a plastic turnstile with a cylindrical core magnet. This kept the forces of attraction constant as the turnstile rotated, lowering friction. And just a minor snap as the teeth turned. The magnetic turnstile approach design worked modestly well, but magnets are dipoles, and forces rise quickly as the gap between the two magnetic disks narrows. A wide gap is advantageous, as it allows for a bit of slop in alignment between the cube and fence. In the end, the lattice is impractically weak. 4 In the video we dubbed the mechanism a "turnstile tesseract". A tesseract is the four-dimensional analog of a three-dimensional cube. The analogy presumes by rotating the turnstiles we are moving through a 4th dimension outside of a pure x,y and z translation. And thus the cube can penetrate a plane.
Mathematicians often solve intractable problems by first increasing dimensionality. Even a simple integral that appears to blow up can often be solved by moving to the complex plane and circling around the singularity onto the imaginary axis. We managed to trisect an angle with only a compass and a straight edge by moving into the third dimension. Slightly cheating by warping the original conditions of this impossible task. Though the solution is amusing and informative. And then there is Sheldon Cooper (Big Bang S3E18) solving an important problem in topology: 5 A year after (re)inventing this mechanism I discovered (upon re-reading "Surely You're Joking, Mr. Feynman") that the turnstile is also called a "shaft passer". 6 James Gleick reminds me of the book Le Passe-muraille (The passer through walls)
|
Contact Greg Blonder by email here - Modified Genuine Ideas, LLC. |